According to the Maximum power transform theorem maximum power is delivered by the load resistance RL when internal resistance of the circuit is equal to the load resistance.
Therefore the internal resistance or equivalent resistance of the Fig. 21 from the load side is
Rth=(4×8)/(4+8)=2.67 Ω= RL
The current follow through the circuit I=24/12=2 Amp,
The open circuit voltage across the load resistance is equal voltage drop across the 8 Ω resistance.
Vo.c=2×8=16 V
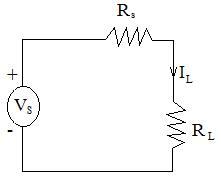
Fig. 21(a)
Here Vo.c=Vs, Rth=Rs=RL=2.67 Ω
The maximum power is delivered from the load resistance
PL=I2L RL=(VS/(RL+RS ))2 RL=(16/5.34)2×2.67=24.03 Watts


 Fig.23
Fig.23